Complexity Explorer Santa Few Institute

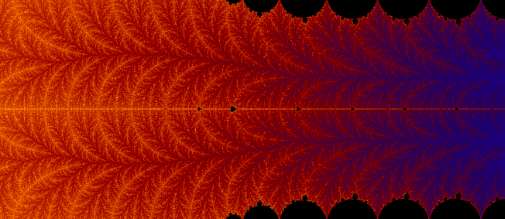
Universal ratios discovered from bifurcation patterns occurring in one-dimensional function maps (such as the logistic map) with a single quadratic maximum, or 'hump'. The bifurcations relate to phenomena with oscillatory (cyclic) behavior, such as swinging pendulums or heart rhythms. The most well-known one, Feigenbaum's Delta, refers to the spacing between parameter values required to double the cycle's length, which decreases exponentially by a factor approaching approximately 4.669. The slightly less well-known Feigenbaum's Alpha refers to the scaling factor by which the x-values decrease for each periodic doubling on said function map, which approaches approximately 2.503.